Having trouble with an oscillating integrating process? Have too much variability in a self-regulated process? Need a refresher about the essentials of Lambda (λ) tuning? Then check out James Beall‘s workshop at the Jan. 21–23 Texas A&M University 2014 Instrumentation Symposium.
James was kind enough to give me a sneak peek at his presentation, and I’ll pass on a few nuggets below. If you find yourself wanting more, be sure to catch his “Model Based Lambda Loop Tuning Methodology” workshop live on Jan. 23 at 1:30. Using examples from two process models that can use Lambda tuning (of the 11 possible), he’ll go over the main elements of Lambda tuning, and talk about common variations and where you’re most likely to encounter them in the field.
After a rundown of a few other PID loop tuning methods—from 1942’s Ziegler and Nichols quarter-amplitude damping to “tune by feel”—James begins by talking about measuring the appropriate process parameters and using those to calculate tuning constants for different types of loops.
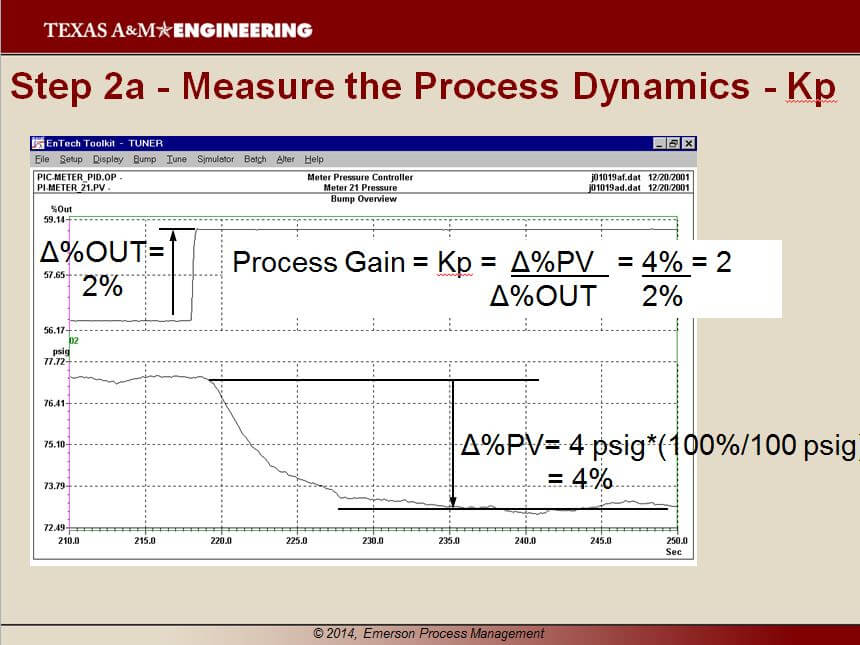
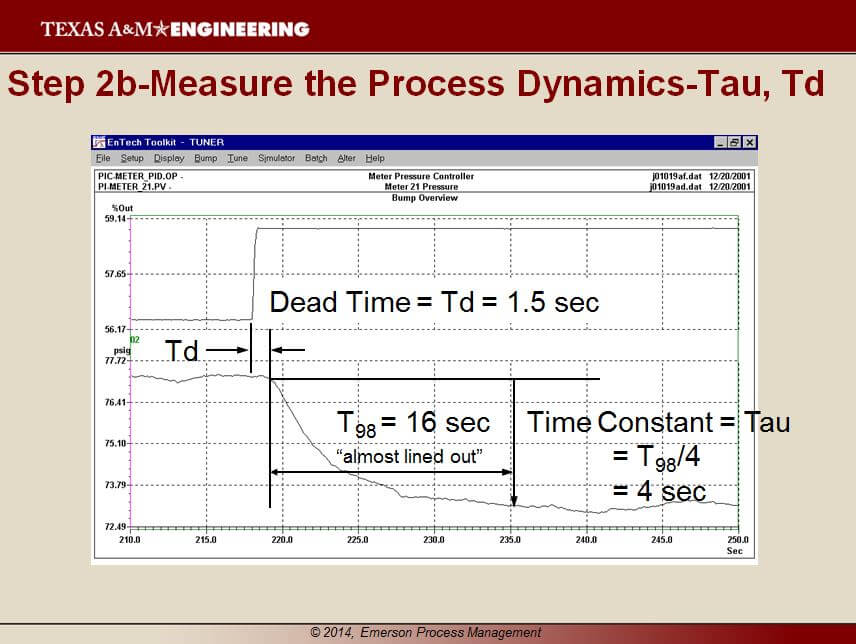
For the basic tuning of a self-regulating loop—approximated with a first-order-plus-dead-time process model—the first step is to perform an open-loop step test. Next, obtain the essential parameters beginning with the following steps, from James’s slides (click to enlarge):
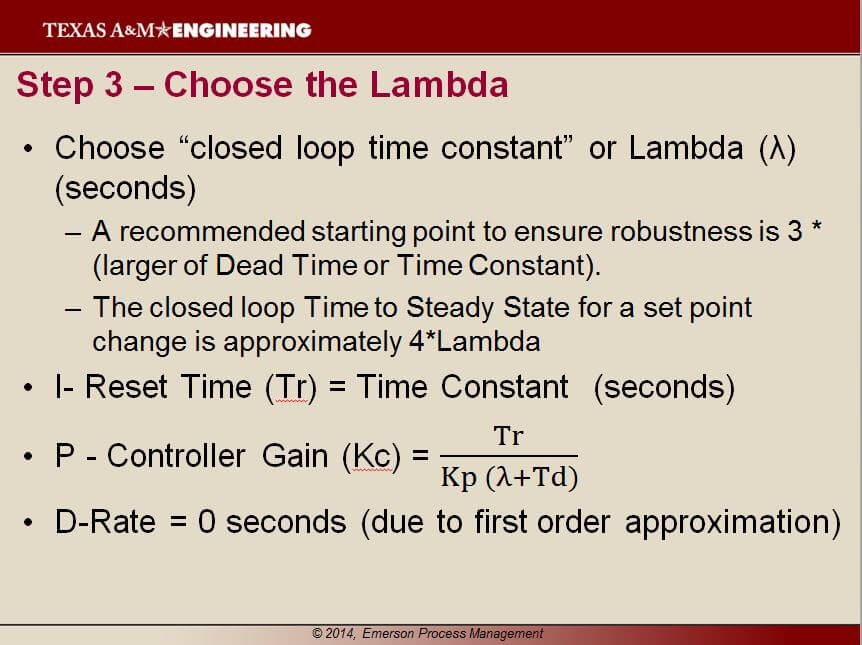
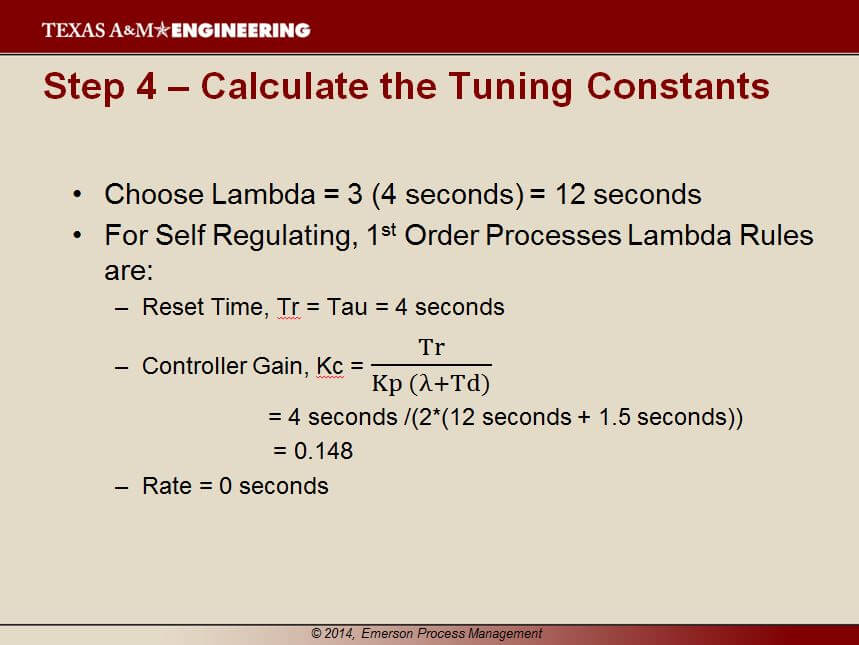
When you have those values in hand, calculating controller gain (Kc) is a breeze, beginning with your choice of lambda. That choice depends on several factors, but a common starting place is about 3 * (the larger of dead time [Td] or the time constant [Tr]) in the standard equation:
Kc = Tr/(Kp*[λ+Td])
Where Tr = Tau
Below, you’ll see two of James’s slides with notes about calculating tuning values. (Click them to enlarge.)
Then test your tuning by putting the process under closed-loop control and making a set-point change. If you chose an appropriate lambda, the process variable won’t overshoot your set point—assuming that’s the result you were aiming for. Choose a very short lambda, and you’ll get a less robust loop and some oscillation around the set point. In general, it should take about 4λ for a Lambda-tuned self-regulated process to reach a new set point.
You’ll also find a good explanation of how to apply Lambda tuning to solve specific problems in this pdf by Emerson Senior Process Control Consultant Mark Coughran, “Lambda Tuning—the Universal Method for PID Controllers in Process Control.” And here’s a good basic tutorial from Control Engineering magazine.
Back to James. His talk next expands into how to modify the basic Lambda tuning method for different situations, with a few example problems of each:
- Self-regulating, second-order over-damped loops.
- Loops controlling integrating processes, in which the process variable doesn’t self-regulate after controller output changes, but keeps moving.
- Attenuating and absorbing variability, especially using level control
For attenuating variability, lambda has to be long enough (that is, the control has to be slow enough) to absorb process variability—but not so long that the level goes beyond an allowable parameter. Choosing lambda in that case involves this equation:
λ = (2*AD)/(Kp*MLD)
Where:
AD = Allowable Level Variation
Kp = Integrating process gain
MLD = Maximum Load Disturbance (in % of controller output)
He’ll also talk about how to properly coordinate multiple interacting loops and minimizing “disturbance amplification.” But for the full treatment, be sure to catch James live in late January.